Base ten blocks first appeared in elementary school classrooms over 50 years ago, when the New Math Movement in the 1960s pushed for a greater conceptual focus in elementary math instruction (Kim & Albert 2014). Teachers have been using them ever since to teach number sense, counting, place value, and whole number and decimal operations. Today, the Common Core Learning Standards delicate one of five learning domains to “Numbers and Operations in Base Ten” in Kindergarten through Grade 5, so base ten blocks are a more important part of elementary math instruction than ever. This article address how base ten blocks can build understanding of place value concepts and skills in the upper elementary grades.

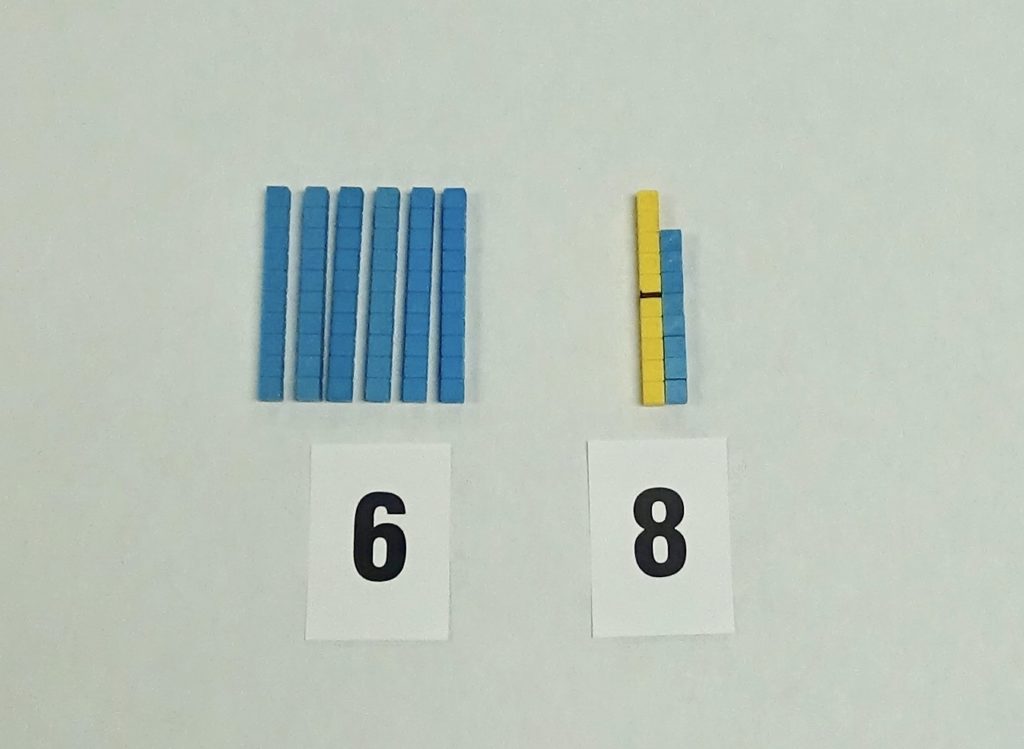

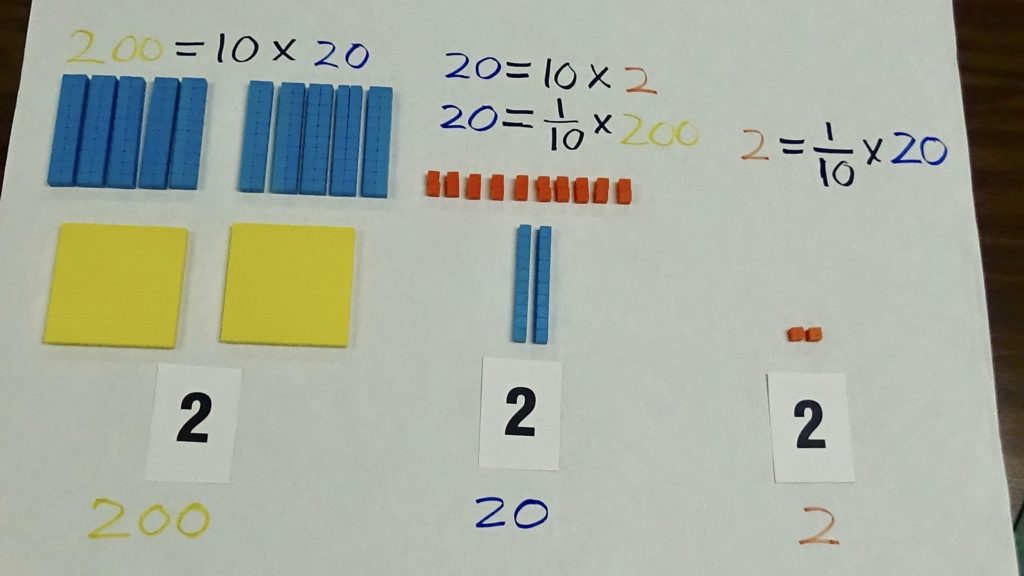
Rounding numbers is a more advanced place value skill that is introduced in third grade and continues in 4th and 5th Grades. Teachers often use a number line to demonstrate this concept/skill, but base ten blocks also work very well in conjunction with numberlines. Take for example, rounding 68 to the nearest ten (represented with base ten blocks – 6 longs and 8 units). The teacher explains that rounding to the nearest ten means expressing the number as the nearest multiple of ten to the original number. The rounded number will either be the exact number of tens (longs), in the original number (6 in this example) or one more ten (long) than the original number (7 in this example) depending on the number of ones (units) in the original number (less than half of a long, 1-4 units – closer to original number of tens; half or more than half a long, 5-9 units- closer to original number of tens (longs) plus one more ten (long). Use a long, with a line drawn splitting it in half, as a reference tool. If the units are less than half of the reference long, push the units and the reference long aside. If the units are equal or greater than half of the reference long, push the units aside and add the reference long to the other longs. The 8 ones (units) in 68 are more than half of ten (a long), so 68 is closer to 7 tens (longs) or 70 than it is to 6 tens (longs) or 60. Teachers and students can continue to demonstrate rounding bigger numbers using base ten blocks or base ten block notation as they progress in through Grades 4 and 5.
Having students imagine that the unit is magnified 1000x so that it looks like the 1000 block, allows students to use the flat as a tenth, a long as a hundredth, and a unit as a thousandth.
In 4th and 5th grade, student are expected to recognize that a digit in one place represents 10 times as much as it represents in the place to its right and 1/10 of what it represents to its left. Take for example the number 222. The digit 2 in the tens place is 10 times as much as the value of the 2 in the ones place. A teacher can demonstrate this by showing that 2 longs (20) are 10 times as much as 2 units (2); 20 = 10 X 2.. More over, the 2 in the tens place is 1/10 as much as the 2 in the hundreds place. A teacher can demonstrate this by showing that 2 longs (20) is 1/10 as much as 2 flats (200); 20 = 1/10 X 200.
The author of GoodNeighborsMath.com has created tools to assist students in identifying and producing the names of large whole number place values and decimal place values. There are free posters, card decks and worksheets to teach and reinforce mnemonic aides.



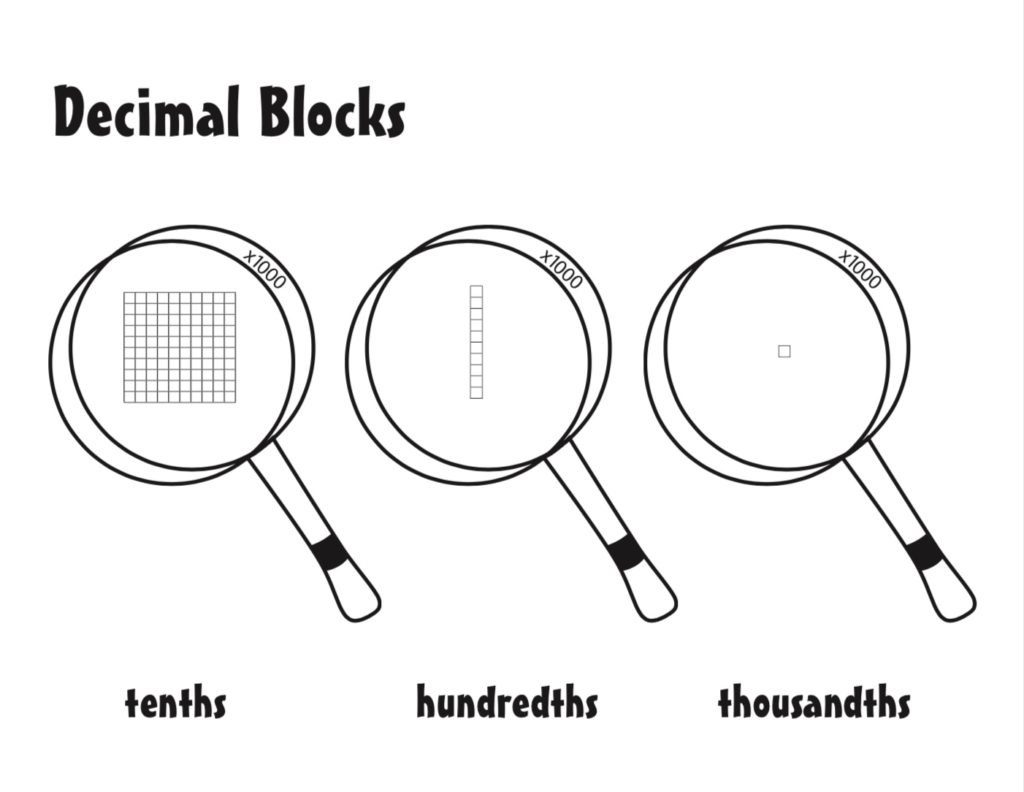
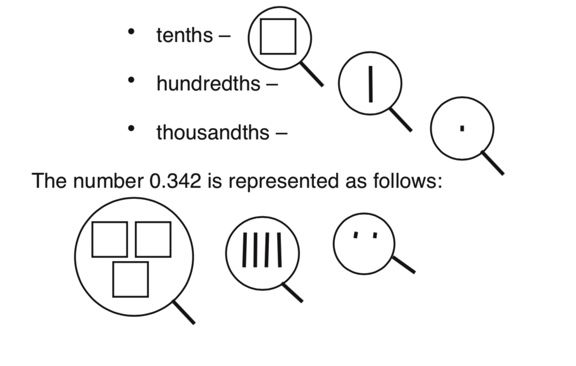
The last place value concepts taught in the upper elementary grades are decimal place values. Although there are no decimal base ten blocks that proportionally correspond with the traditional base ten blocks, base ten blocks can still be used effectively with decimal concepts and skills. Having students imagine that the unit is magnified 1000x so that it looks like the 1000 block, allows students to use the flat as a tenth, a long as a hundredth, and a unit as a thousandth. There are excellent reference drawings of the decimal base ten blocks, descriptions of decimal base ten block notation, and a decimal card deck in The Good Neighbors Upper Elementary Backline Masters. Comparing decimal numbers can be difficult for children, so making the numbers with base ten blocks or drawing them with base ten block notation is essential for conceptual understanding.
Place value concepts and skills are the foundation for much of the math curriculum of the upper elementary grades. Base ten blocks, and base ten block notation provide an invaluable model for students to visualize these concepts and skills.
References
Kim, R. & Albert, L. (2014) The history of base-ten-blocks: Why and who made base-ten-blocks? eScholarship@BC Boston College University Libraries.