Introduction
Upper elementary students learn the standard algorithm for subtraction at some point during their elementary math instruction. It is generally the most efficient computational method, and it is essential for efficient division computations later on in the curriculum. Teachers often introduce the algorithm conceptually by demonstrating the process with base ten blocks. Next, students solve subtraction computations pictorially using base ten block notation. Once students have success subtracting with pictures, teachers naturally transition to having students subtract with numbers.
The leap from the concrete pictorial demonstration, to the abstract numeric demonstration of the algorithm is greater than most teachers think. It is for this reason that students often have difficulty learning and retaining the process of the standard algorithm with numbers. I know from my experience as a Special Ed. Teacher and Math Interventionist, the number of upper elementary students who need remediation/intervention for subtraction computations is exorbitant.
The Missing Step – The Bridge Connecting Concrete and Abstract
To bridge the gap between the concrete and the abstract, the ‘Hybrid Method’ for subtraction based on the picture book, The Good Neighbors’ Cheese Feast was created. This novel intervention incorporates digit/place value cards, recipe cards, and thumbnail illustrations to make the conceptual elements of the algorithm visible for students. The ‘Hybrid Method’ is Step 4 of “The 5 ‘Novel’ Steps to Teach Subtraction with Regrouping – Conceptually”.

The ‘Hybrid Method’ is based on The Good Neighbors’ Cheese Feast.






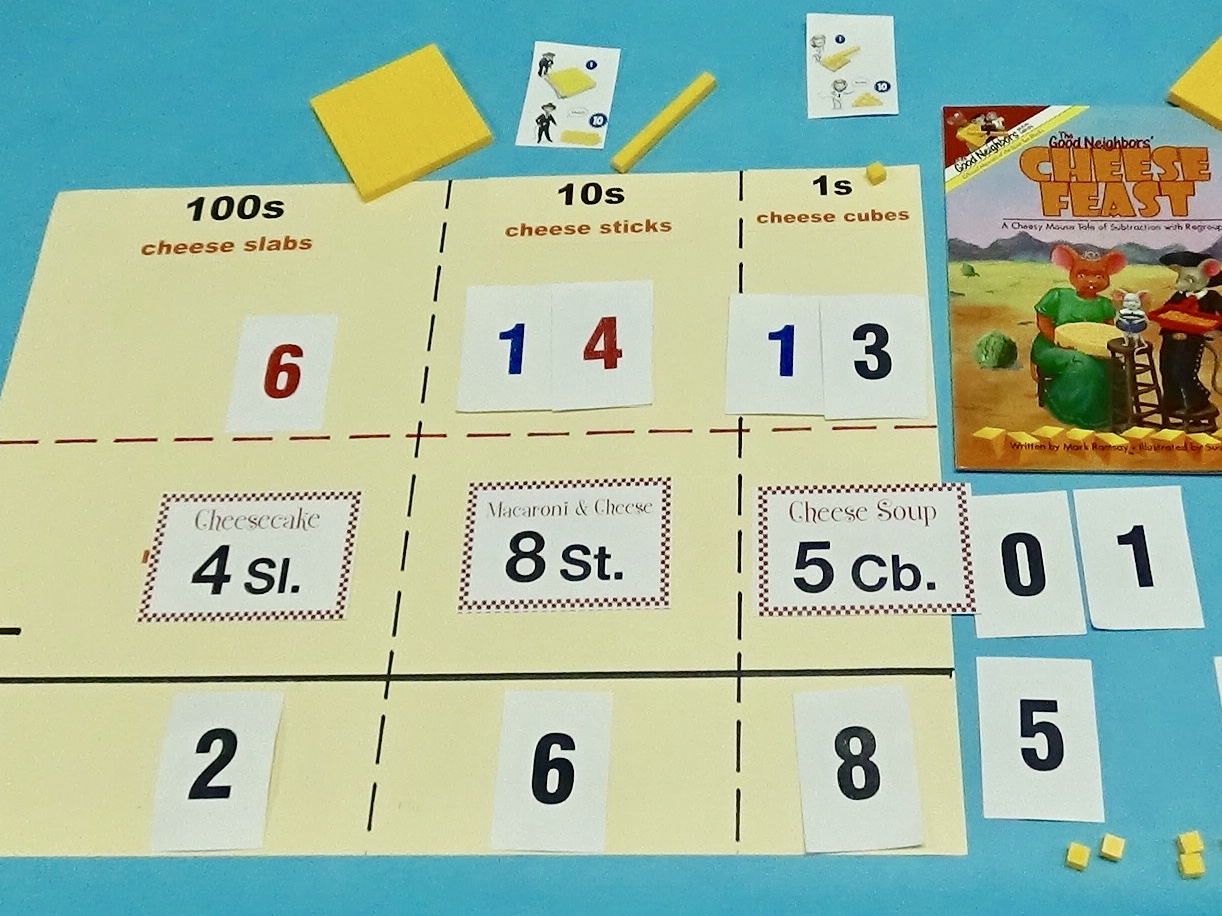
The cards are the key to the ‘Hybrid Method’. The number cards 0-9 have black digits on one side and red digits one less on the other side. This allows students to change a number to one less by flipping it over (ie. 8 flipped over to 7). The blue 10 cards allow students to make the numbers 10-19 conceptually (ie.13 is made with the 10 card and the 3 card, 10+3=13, not a 1 card and a 3 card, 1+3=4). The two thumbnail illustration cards show the two different regrouping processes from the story. Each card shows the character cutting one of his neighbor’s sized block of cheese into 10 of his sized blocks of cheese (base ten block visual models). The recipe cards as minuend establishes the ‘Cooking/Baking Schema for Subtraction’. The minuend is clearly defined for students and it is clearly distinguished from the subtrahend (a common misunderstanding of students).
When the cards are used in conjunction with each other, the subtraction with regrouping process comes alive. The regrouping process with the “Hybrid Method’ involves flipping over the digit card and the 10 card/thumbnail card combo of the next bigger place value. The flipping results in regrouping the original digit to a digit one less than the original digit and 1 with a thumbnail picture next to it (ie, 8 is flipped to 7 and 1/thumbnail picture). It is worthwhile to have students linger on this step. The 1/thumbnail picture is given (slid) to the next smaller place value where it is turned into 10 smaller units by removing the thumbnail picture and revealing the zero. The action of removing the thumbnail makes the illustration on the thumbnail come to fruition – 1 bigger unit was changed to 10 smaller units. Another step that students should linger on. The newly acquired 10 units and the original units can easily be combined by placing the single digit on top of the 0 of the 10 card. Then students can perform the subtraction fact for that given place value and the subtraction process continues on to the next place value(s) until the algorithm is complete. Students are encouraged to vocalize their actions as they perform the ‘Hybrid Method’ to further ingrain the process in their minds.
The action of removing the thumbnail makes the illustration on the thumbnail come to fruition – 1 bigger unit was changed to 10 smaller units. Another step that students should linger on.
Conclusion
The ‘Hybrid Method’ combines visual, kinesthetic, numeric, and auditory/verbal modalities to provide an extraordinary learning expense for students. Because the ‘Hybrid Method’ is so effective, student generally need only a few times to perform it before they have a lasting conceptual understanding and can apply it to paper/pencil computations. The ‘Hybrid Method’ until now has been the missing step teachers needed to connect concrete and abstract understanding of subtraction with regrouping and the standard algorithm.

